Average level

Right triangle. The Complete Illustrated Guide (2019)

RIGHT TRIANGLE. FIRST LEVEL.

In problems, the right angle is not at all necessary - the lower left, so you need to learn to recognize a right triangle in this form,

and in this

and in this

What's good about a right triangle? Well... first of all, there are special beautiful names for his sides.

Attention to the drawing!

Remember and don't confuse: there are two legs, and there is only one hypotenuse(one and only, unique and longest)!

Well, we’ve discussed the names, now the most important thing: the Pythagorean Theorem.

Pythagorean theorem.

This theorem is the key to solving many problems involving a right triangle. Pythagoras proved it completely time immemorial, and since then she has brought a lot of benefit to those who know her. And the best thing about it is that it is simple.

So, Pythagorean theorem:

Do you remember the joke: “Pythagorean pants are equal on all sides!”?

Let's draw these same Pythagorean pants and look at them.

Doesn't it look like some kind of shorts? Well, on which sides and where are they equal? Why and where did the joke come from? And this joke is connected precisely with the Pythagorean theorem, or more precisely with the way Pythagoras himself formulated his theorem. And he formulated it like this:

"Sum areas of squares, built on the legs, is equal to square area, built on the hypotenuse."

Does it really sound a little different? And so, when Pythagoras drew the statement of his theorem, this is exactly the picture that came out.

In this picture, the sum of the areas of the small squares is equal to the area of ​​the large square. And so that children can better remember that the sum of the squares of the legs is equal to the square of the hypotenuse, someone witty came up with this joke about Pythagorean pants.

Why are we now formulating the Pythagorean theorem?

Did Pythagoras suffer and talk about squares?

You see, in ancient times there was no... algebra! There were no signs and so on. There were no inscriptions. Can you imagine how terrible it was for the poor ancient students to remember everything in words??! And we can rejoice that we have a simple formulation of the Pythagorean theorem. Let's repeat it again to remember it better:

It should be easy now:

Square of the hypotenuse equal to the sum squares of legs.

Well, the most important theorem about right triangles has been discussed. If you are interested in how it is proven, read the following levels of theory, and now let's move on... to dark forest... trigonometry! To the terrible words sine, cosine, tangent and cotangent.

Sine, cosine, tangent, cotangent in a right triangle.

In fact, everything is not so scary at all. Of course, the “real” definition of sine, cosine, tangent and cotangent should be looked at in the article. But I really don’t want to, do I? We can rejoice: to solve problems about a right triangle, you can simply fill in the following simple things:

Why is everything just about the corner? Where is the corner? In order to understand this, you need to know how statements 1 - 4 are written in words. Look, understand and remember!

1.
Actually it sounds like this:

What about the angle? Is there a leg that is opposite the corner, that is, an opposite (for an angle) leg? Of course have! This is a leg!

What about the angle? Look carefully. Which leg is adjacent to the corner? Of course, the leg. This means that for the angle the leg is adjacent, and

Now, pay attention! Look what we got:

See how cool it is:

Now let's move on to tangent and cotangent.

How can I write this down in words now? What is the leg in relation to the angle? Opposite, of course - it “lies” opposite the corner. What about the leg? Adjacent to the corner. So what have we got?

See how the numerator and denominator have swapped places?

And now the corners again and made an exchange:

Summary

Let's briefly write down everything we've learned.

Pythagorean theorem:

The main theorem about right triangles is the Pythagorean theorem.

Pythagorean theorem

By the way, do you remember well what legs and hypotenuse are? If not very good, then look at the picture - refresh your knowledge

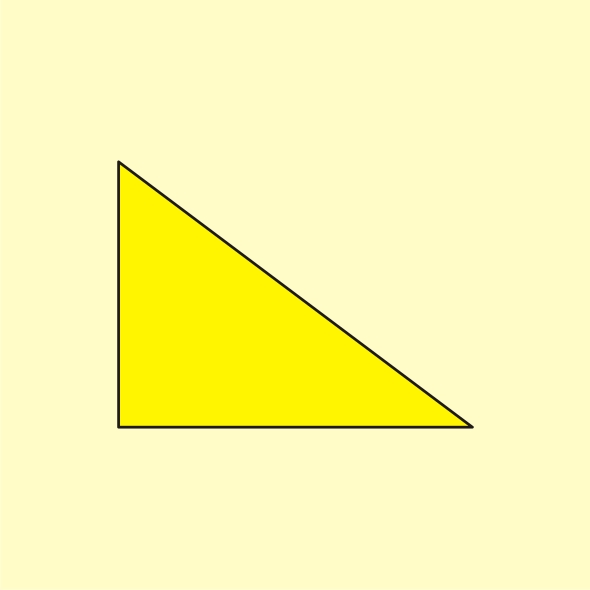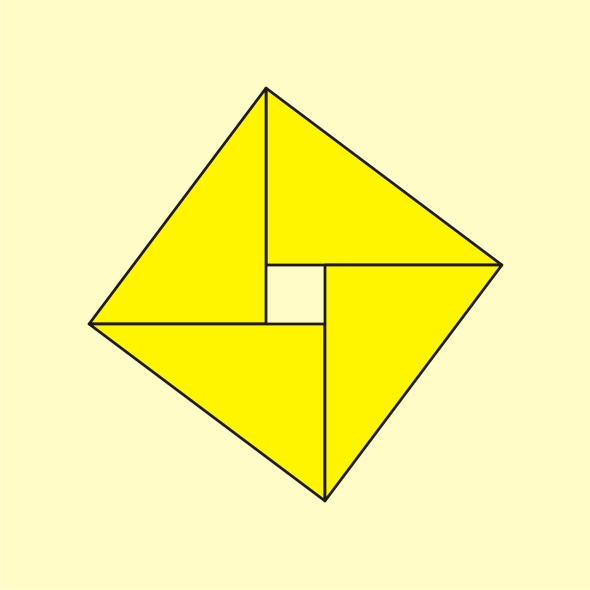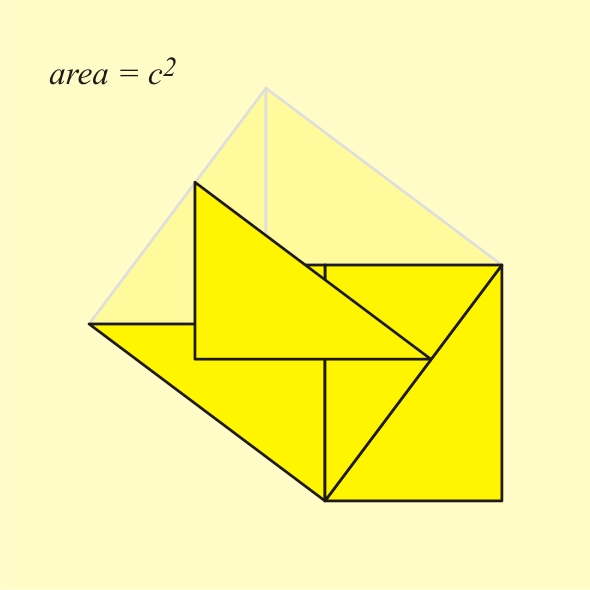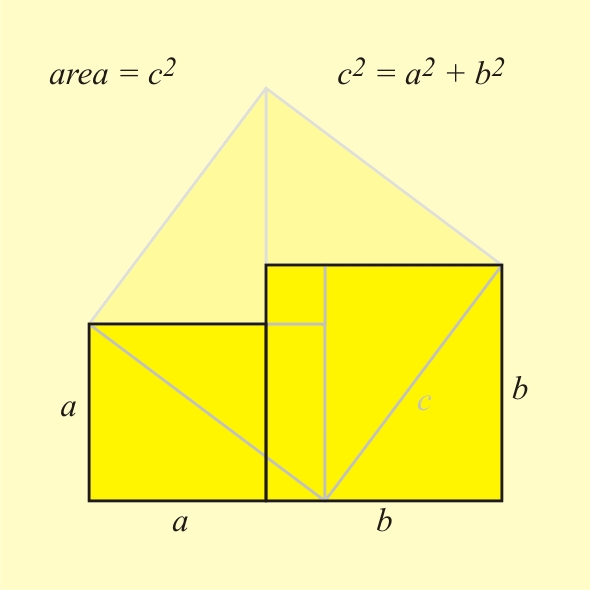
It is quite possible that you have already used the Pythagorean theorem many times, but have you ever wondered why such a theorem is true? How can I prove it? Let's do like the ancient Greeks. Let's draw a square with a side.

See how cleverly we divided its sides into lengths and!

Now let's connect the marked dots

Here we, however, noted something else, but you yourself look at the drawing and think why this is so.

What is the area of ​​the larger square? Right, . What about a smaller area? Certainly, . The total area of ​​the four corners remains. Imagine that we took them two at a time and leaned them against each other with their hypotenuses. What happened? Two rectangles. This means that the area of ​​the “cuts” is equal.

Let's put it all together now.

Let's transform:

So we visited Pythagoras - we proved his theorem in an ancient way.

Right triangle and trigonometry

For a right triangle, the following relations hold:

Sine of an acute angle equal to the ratio opposite side to the hypotenuse

The cosine of an acute angle is equal to the ratio adjacent leg to the hypotenuse.

The tangent of an acute angle is equal to the ratio of the opposite side to the adjacent side.

The cotangent of an acute angle is equal to the ratio of the adjacent side to the opposite side.

And once again all this in the form of a tablet:

It is very comfortable!

Signs of equality of right triangles

I. On two sides

II. By leg and hypotenuse

III. By hypotenuse and acute angle

IV. Along the leg and acute angle

a)

b)

Attention! It is very important here that the legs are “appropriate”. For example, if it goes like this:

THEN TRIANGLES ARE NOT EQUAL, despite the fact that they have one identical acute angle.

Need to in both triangles the leg was adjacent, or in both it was opposite.

Have you noticed how the signs of equality of right triangles differ from the usual signs of equality of triangles? Take a look at the topic “and pay attention to the fact that for equality of “ordinary” triangles, three of their elements must be equal: two sides and the angle between them, two angles and the side between them, or three sides. But for the equality of right triangles, only two corresponding elements are enough. Great, right?

The situation is approximately the same with the signs of similarity of right triangles.

Signs of similarity of right triangles

I. Along an acute angle

II. On two sides

III. By leg and hypotenuse

Median in a right triangle

Why is this so?

Instead of a right triangle, consider a whole rectangle.

Let's draw a diagonal and consider a point - the point of intersection of the diagonals. What do you know about the diagonals of a rectangle?

And what follows from this?

So it turned out that

1. - median:

Remember this fact! Helps a lot!

What’s even more surprising is that the opposite is also true.

What good can be obtained from the fact that the median drawn to the hypotenuse is equal to half the hypotenuse? Let's look at the picture

Look carefully. We have: , that is, the distances from the point to all three vertices of the triangle turned out to be equal. But there is only one point in the triangle, the distances from which from all three vertices of the triangle are equal, and this is the CENTER OF THE CIRCLE. So what happened?

So let's start with this “besides...”.

Let's look at and.

But similar triangles all angles are equal!

The same can be said about and

Now let's draw it together:

What benefit can be derived from this “triple” similarity?

Well, for example - two formulas for the height of a right triangle.

Let us write down the relations of the corresponding parties:

To find the height, we solve the proportion and get the first formula "Height in a right triangle":

So, let's apply the similarity: .

What will happen now?

Again we solve the proportion and get the second formula:

You need to remember both of these formulas very well and use the one that is more convenient. Let's write them down again

Pythagorean theorem:

In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the legs: .

Signs of equality of right triangles:

• on two sides:
• by leg and hypotenuse: or
• along the leg and adjacent acute angle: or
• along the leg and the opposite acute angle: or
• by hypotenuse and acute angle: or.

Signs of similarity of right triangles:

• one acute corner: or
• from the proportionality of two legs:
• from the proportionality of the leg and hypotenuse: or.

Sine, cosine, tangent, cotangent in a right triangle

• The sine of an acute angle of a right triangle is the ratio of the opposite side to the hypotenuse:
• The cosine of an acute angle of a right triangle is the ratio of the adjacent leg to the hypotenuse:
• The tangent of an acute angle of a right triangle is the ratio of the opposite side to the adjacent side:
• The cotangent of an acute angle of a right triangle is the ratio of the adjacent side to the opposite side: .

Height of a right triangle: or.

In a right triangle, the median drawn from the vertex right angle, is equal to half the hypotenuse: .

Area of ​​a right triangle:

• via legs:

Pythagorean theorem: Sum of areas of squares resting on legs ( a And b), equal to the area of ​​the square built on the hypotenuse ( c).

Geometric formulation:

The theorem was originally formulated as follows:

Algebraic formulation:

That is, denoting the length of the hypotenuse of the triangle by c, and the lengths of the legs through a And b :

a 2 + b 2 = c 2

Both formulations of the theorem are equivalent, but the second formulation is more elementary; it does not require the concept of area. That is, the second statement can be verified without knowing anything about the area and by measuring only the lengths of the sides of a right triangle.

Converse Pythagorean theorem:

Proof

On this moment V scientific literature 367 proofs of this theorem have been recorded. Probably, the Pythagorean theorem is the only theorem with such an impressive number of proofs. Such diversity can only be explained by the fundamental significance of the theorem for geometry.

Of course, conceptually all of them can be divided into a small number of classes. The most famous of them: proofs by the area method, axiomatic and exotic proofs (for example, using differential equations).

Through similar triangles

The following proof of the algebraic formulation is the simplest of the proofs, constructed directly from the axioms. In particular, it does not use the concept of area of ​​a figure.

Let ABC there is a right triangle with a right angle C. Let's draw the height from C and denote its base by H. Triangle ACH similar to a triangle ABC at two corners. Likewise, triangle CBH similar ABC. By introducing the notation

we get

What is equivalent

Adding it up, we get

Proofs using the area method

The proofs below, despite their apparent simplicity, are not so simple at all. They all use properties of area, the proofs of which more difficult proof the Pythagorean theorem itself.

Proof via equicomplementation

1. Let's arrange four equal right triangle as shown in Figure 1.
2. Quadrangle with sides c is a square, since the sum of two sharp corners 90°, and the unfolded angle is 180°.
3. The area of ​​the entire figure is equal, on the one hand, to the area of ​​a square with side (a + b), and on the other hand, to the sum four squares triangles and two inner squares.

Q.E.D.

Proofs through equivalence

Elegant proof using permutation

An example of one such proof is shown in the drawing on the right, where a square built on the hypotenuse is rearranged into two squares built on the legs.

Euclid's proof

Drawing for Euclid's proof

Illustration for Euclid's proof

The idea of ​​Euclid's proof is as follows: let's try to prove that half the area of ​​the square built on the hypotenuse is equal to the sum of the half areas of the squares built on the legs, and then the areas of the large and two small squares are equal.

Let's look at the drawing on the left. On it we constructed squares on the sides of a right triangle and drew a ray s from the vertex of the right angle C perpendicular to the hypotenuse AB, it cuts the square ABIK, built on the hypotenuse, into two rectangles - BHJI and HAKJ, respectively. It turns out that the areas of these rectangles are exactly equal to the areas of the squares built on the corresponding legs.

Let's try to prove that the area of ​​the square DECA is equal to the area of ​​the rectangle AHJK. To do this, we will use an auxiliary observation: The area of ​​a triangle with the same height and base as given rectangle, equal to half the area of ​​the given rectangle. This is a consequence of defining the area of ​​a triangle as half the product of the base and the height. From this observation it follows that the area of ​​triangle ACK is equal to the area of ​​triangle AHK (not shown in the figure), which in turn is equal to half the area of ​​rectangle AHJK.

Let us now prove that the area of ​​triangle ACK is also equal to half the area of ​​square DECA. The only thing that needs to be done for this is to prove the equality of triangles ACK and BDA (since the area of ​​triangle BDA is equal to half the area of ​​the square according to the above property). The equality is obvious, the triangles are equal on both sides and the angle between them. Namely - AB=AK,AD=AC - the equality of the angles CAK and BAD is easy to prove by the method of motion: we rotate the triangle CAK 90° counterclockwise, then it is obvious that the corresponding sides of the two triangles in question will coincide (due to the fact that the angle at the vertex of the square is 90°).

The reasoning for the equality of the areas of the square BCFG and the rectangle BHJI is completely similar.

Thus, we proved that the area of ​​a square built on the hypotenuse is composed of the areas of squares built on the legs. The idea behind this proof is further illustrated by the animation above.

Proof of Leonardo da Vinci

Proof of Leonardo da Vinci

The main elements of the proof are symmetry and motion.

Let's consider the drawing, as can be seen from the symmetry, a segment CI cuts the square ABHJ into two identical parts (since triangles ABC And JHI equal in construction). Using a 90 degree counterclockwise rotation, we see the equality of the shaded figures CAJI And GDAB . Now it is clear that the area of ​​the figure we have shaded is equal to the sum of half the areas of the squares built on the legs and the area of ​​the original triangle. On the other hand, it is equal to half the area of ​​the square built on the hypotenuse, plus the area of ​​the original triangle. The last step in the proof is left to the reader.

Proof by the infinitesimal method

The following proof using differential equations is often attributed to the famous English mathematician Hardy, who lived in the first half of the 20th century.

Looking at the drawing shown in the figure and observing the change in side a, we can write the following relation for infinitesimal side increments With And a(using triangle similarity):

Proof by the infinitesimal method

Using the method of separation of variables, we find

More general expression to change the hypotenuse in case of increments of both legs

Integrating given equation and using initial conditions, we get

c 2 = a 2 + b 2 + constant.

Thus we arrive at the desired answer

c 2 = a 2 + b 2 .

How easy it is to see quadratic dependence appears in the final formula thanks to linear proportionality between the sides of the triangle and the increments, while the sum is associated with independent contributions from the increment of different legs.

A simpler proof can be obtained if we assume that one of the legs does not experience an increase (in in this case leg b). Then for the integration constant we obtain

Variations and generalizations

• If instead of squares we construct other similar figures on the sides, then the following generalization of the Pythagorean theorem is true: In a right triangle, the sum of the areas is similar figures built on the legs is equal to the area of ​​the figure built on the hypotenuse. In particular:
  • The sum of the areas of regular triangles built on the sides is equal to the area regular triangle, built on the hypotenuse.
  • The sum of the areas of semicircles built on the legs (as on the diameter) is equal to the area of ​​the semicircle built on the hypotenuse. This example is used to prove the properties of figures bounded by the arcs of two circles and called Hippocratic lunulae.

Story

Chu-pei 500–200 BC. On the left is the inscription: the sum of the squares of the lengths of the height and base is the square of the length of the hypotenuse.

The ancient Chinese book Chu-pei talks about Pythagorean triangle with sides 3, 4 and 5: In the same book, a drawing is proposed that coincides with one of the drawings of the Hindu geometry of Bashara.

Cantor (the greatest German historian of mathematics) believes that the equality 3² + 4² = 5² was already known to the Egyptians around 2300 BC. e., during the time of King Amenemhat I (according to papyrus 6619 of the Berlin Museum). According to Cantor, the harpedonaptes, or "rope pullers", built right angles using right triangles with sides of 3, 4 and 5.

It is very easy to reproduce their method of construction. Let's take a rope 12 m long and tie a colored strip to it at a distance of 3 m. from one end and 4 meters from the other. The right angle will be enclosed between sides 3 and 4 meters long. It could be objected to the Harpedonaptians that their method of construction becomes superfluous if one uses, for example, a wooden square, which is used by all carpenters. Indeed, Egyptian drawings are known in which such a tool is found, for example, drawings depicting a carpenter's workshop.

Somewhat more is known about the Pythagorean theorem among the Babylonians. In one text dating back to the time of Hammurabi, that is, to 2000 BC. e., an approximate calculation of the hypotenuse of a right triangle is given. From this we can conclude that in Mesopotamia they were able to perform calculations with right triangles, at least in some cases. Based, on the one hand, on the current level of knowledge about Egyptian and Babylonian mathematics, and on the other, on a critical study of Greek sources, Van der Waerden (Dutch mathematician) came to the following conclusion:

Literature

In Russian

• Skopets Z. A. Geometric miniatures. M., 1990
• Elensky Shch. In the footsteps of Pythagoras. M., 1961
• Van der Waerden B. L. Awakening Science. Mathematics Ancient Egypt, Babylon and Greece. M., 1959
• Glazer G.I. History of mathematics at school. M., 1982
• W. Litzman, “The Pythagorean Theorem” M., 1960.
  • A site about the Pythagorean theorem with a large number of proofs, material taken from the book by V. Litzmann, a large number of drawings are presented in the form of separate graphic files.
• The Pythagorean theorem and Pythagorean triples chapter from the book by D. V. Anosov “A look at mathematics and something from it”
• About the Pythagorean theorem and methods of proving it G. Glaser, academician of the Russian Academy of Education, Moscow

In English

• Pythagorean Theorem at WolframMathWorld
• Cut-The-Knot, section on the Pythagorean theorem, about 70 proofs and extensive additional information (English)

Wikimedia Foundation. 2010.

Make sure that the triangle you are given is a right triangle, as the Pythagorean Theorem only applies to right triangles. In right triangles, one of the three angles is always 90 degrees.

• A right angle in a right triangle is indicated by a square icon rather than the curve that represents oblique angles.

Label the sides of the triangle. Label the legs as “a” and “b” (legs are sides intersecting at right angles), and the hypotenuse as “c” (the hypotenuse is the most big side right triangle, lying opposite the right angle).

Determine which side of the triangle you want to find. The Pythagorean theorem allows you to find any side of a right triangle (if the other two sides are known). Determine which side (a, b, c) you need to find.

• For example, given a hypotenuse equal to 5, and given a leg equal to 3. In this case, it is necessary to find the second leg. We'll come back to this example later.
• If the other two sides are unknown, you need to find the length of one of the unknown sides to be able to apply the Pythagorean theorem. To do this, use the basic trigonometric functions(if you are given the value of one of the oblique angles).

Substitute the values ​​given to you (or the values ​​you found) into the formula a 2 + b 2 = c 2. Remember that a and b are legs, and c is the hypotenuse.

• In our example, write: 3² + b² = 5².

Square each known side. Or leave the powers - you can square the numbers later.

• In our example, write: 9 + b² = 25.

Isolate the unknown side on one side of the equation. To do this, move known values to the other side of the equation. If you find the hypotenuse, then in the Pythagorean theorem it is already isolated on one side of the equation (so you don't need to do anything).

• In our example, move 9 to right side equations to isolate the unknown b². You will get b² = 16.

Remove Square root from both sides of the equation after the unknown (squared) is present on one side of the equation and the free term (number) is present on the other side.

• In our example, b² = 16. Take the square root of both sides of the equation and get b = 4. Thus, the second leg is 4.

Use the Pythagorean theorem in Everyday life, since it can be used in large number practical situations. To do this, learn to recognize right triangles in everyday life - in any situation in which two objects (or lines) intersect at right angles, and a third object (or line) connects (diagonally) the tops of the first two objects (or lines), you can use the Pythagorean theorem to find the unknown side (if the other two sides are known).

• Example: given a staircase leaning against a building. Bottom part The stairs are located 5 meters from the base of the wall. Top part The stairs are located 20 meters from the ground (up the wall). What is the length of the stairs?
  • “5 meters from the base of the wall” means that a = 5; “located 20 meters from the ground” means that b = 20 (that is, you are given two legs of a right triangle, since the wall of the building and the surface of the Earth intersect at right angles). The length of the staircase is the length of the hypotenuse, which is unknown.
    • a² + b² = c²
    • (5)² + (20)² = c²
    • 25 + 400 = c²
    • 425 = c²
    • c = √425
    • c = 20.6. Thus, the approximate length of the stairs is 20.6 meters.

MEASUREMENT OF AREA OF GEOMETRIC FIGURES.

§ 58. PYTHAGOREAN THEOREM 1.

__________
1 Pythagoras is a Greek scientist who lived about 2500 years ago (564-473 BC).
_________

Let us be given a right triangle whose sides A, b And With(drawing 267).

Let's build squares on its sides. The areas of these squares are respectively equal A 2 , b 2 and With 2. Let's prove that With 2 = a 2 + b 2 .

Let's construct two squares MKOR and M"K"O"R" (drawings 268, 269), taking as the side of each of them a segment equal to the sum of the legs of the right triangle ABC.

Having completed the constructions shown in drawings 268 and 269 in these squares, we will see that the MCOR square is divided into two squares with areas A 2 and b 2 and four equal right triangles, each of which is equal to right triangle ABC. The square M"K"O"R" was divided into a quadrangle (it is shaded in drawing 269) and four right triangles, each of which is also equal to triangle ABC. A shaded quadrilateral is a square, since its sides are equal (each is equal to the hypotenuse of triangle ABC, i.e. With), and the angles are right / 1 + / 2 = 90°, from where / 3 = 90°).

Thus, the sum of the areas of the squares built on the legs (in drawing 268 these squares are shaded) is equal to the area of ​​the square MCOR without the sum of the areas of four equal triangles, and the area of ​​the square built on the hypotenuse (in drawing 269 this square is also shaded) is equal to the area of ​​the square M"K"O"R", equal to the square of MCOR, without the sum of the areas of four similar triangles. Therefore, the area of ​​a square built on the hypotenuse of a right triangle is equal to the sum of the areas of the squares built on the legs.

We get the formula With 2 = a 2 + b 2 where With- hypotenuse, A And b- legs of a right triangle.

The Pythagorean theorem is usually formulated briefly as follows:

The square of the hypotenuse of a right triangle is equal to the sum of the squares of the legs.

From the formula With 2 = a 2 + b 2 you can get the following formulas:

A 2 = With 2 - b 2 ;
b 2 = With 2 - A 2 .

These formulas can be used to find unknown side a right triangle along its two given sides.
For example:

a) if the legs are given A= 4 cm, b=3 cm, then you can find the hypotenuse ( With):
With 2 = a 2 + b 2, i.e. With 2 = 4 2 + 3 2 ; with 2 = 25, whence With= √25 =5 (cm);

b) if the hypotenuse is given With= 17 cm and leg A= 8 cm, then you can find another leg ( b):

b 2 = With 2 - A 2, i.e. b 2 = 17 2 - 8 2 ; b 2 = 225, from where b= √225 = 15 (cm).

Consequence: If two right triangles ABC and A have 1 B 1 C 1 hypotenuse With And With 1 are equal, and leg b triangle ABC is longer than the leg b 1 triangle A 1 B 1 C 1,
then the leg A triangle ABC less leg A 1 triangle A 1 B 1 C 1. (Make a drawing illustrating this consequence.)

In fact, based on the Pythagorean theorem we obtain:

A 2 = With 2 - b 2 ,
A 1 2 = With 1 2 - b 1 2

In the written formulas, the minuends are equal, and the subtrahend in the first formula is greater than the subtrahend in the second formula, therefore, the first difference is less than the second,
i.e. A 2 < A 12 . Where A< A 1 .

Exercises.

1. Using drawing 270, prove the Pythagorean theorem for an isosceles right triangle.

2. One leg of a right triangle is 12 cm, the other is 5 cm. Calculate the length of the hypotenuse of this triangle.

3. The hypotenuse of a right triangle is 10 cm, one of the legs is 8 cm. Calculate the length of the other leg of this triangle.

4. The hypotenuse of a right triangle is 37 cm, one of its legs is 35 cm. Calculate the length of the other leg of this triangle.

5. Construct a square with an area twice the size of the given one.

6. Construct a square with an area half the size of the given one. Note. Draw diagonals in this square. The squares constructed on the halves of these diagonals will be the ones we are looking for.

7. The legs of a right triangle are respectively 12 cm and 15 cm. Calculate the length of the hypotenuse of this triangle with an accuracy of 0.1 cm.

8. The hypotenuse of a right triangle is 20 cm, one of its legs is 15 cm. Calculate the length of the other leg to the nearest 0.1 cm.

9. How long must the ladder be so that it can be placed against a window located at a height of 6 m, if the lower end of the ladder must be 2.5 m from the building? (Chart 271.)

home

Methods for proving the Pythagorean theorem.

G. Glaser,
Academician of the Russian Academy of Education, Moscow

About the Pythagorean theorem and methods of proving it

The area of ​​a square built on the hypotenuse of a right triangle is equal to the sum of the areas of the squares built on its legs...

This is one of the most famous geometric theorems ancient times, called the Pythagorean theorem. Almost everyone who has ever studied planimetry knows it even now. It seems to me that if we want to let you know extraterrestrial civilizations about the existence of intelligent life on Earth, then an image of the Pythagorean figure should be sent into space. I think that if thinking beings can accept this information, then without complex signal decoding they will understand that there is a fairly developed civilization on Earth.

The famous Greek philosopher and mathematician Pythagoras of Samos, after whom the theorem is named, lived about 2.5 thousand years ago. Those that have reached us biographical information about Pythagoras are fragmentary and far from reliable. Many legends are associated with his name. It is reliably known that Pythagoras traveled a lot in the countries of the East, visiting Egypt and Babylon. In one of Greek colonies Southern Italy he founded the famous " Pythagorean school", who played important role in scientific and political life ancient Greece. It is Pythagoras who is credited with proving the famous geometric theorem. Based on legends spread famous mathematicians(Proclus, Plutarch, etc.), long time It was believed that this theorem was not known before Pythagoras, hence the name - the Pythagorean theorem.

There is no doubt, however, that this theorem was known many years before Pythagoras. So, 1500 years before Pythagoras, the ancient Egyptians knew that a triangle with sides 3, 4 and 5 is right-angled, and used this property (i.e. the theorem inverse to the Pythagorean theorem) to construct right angles when planning land plots and building structures. Even today, rural builders and carpenters, when laying the foundation of a hut and making its parts, draw this triangle to obtain a right angle. The same thing was done thousands of years ago in the construction of magnificent temples in Egypt, Babylon, China, and probably in Mexico. The oldest Chinese mathematical and astronomical work that has come down to us, Zhou Bi, written about 600 years before Pythagoras, contains, among other proposals related to the right triangle, the Pythagorean theorem. Even earlier this theorem was known to the Hindus. Thus, Pythagoras did not discover this property of a right triangle; he was probably the first to generalize and prove it, thereby transferring it from the field of practice to the field of science. We don't know how he did it. Some historians of mathematics assume that Pythagoras’s proof was not fundamental, but only a confirmation, a test of this property on a number of particular types of triangles, starting with an isosceles right triangle, for which it obviously follows from Fig. 1.

WITH Since ancient times, mathematicians have found more and more new proofs of the Pythagorean theorem, more and more new ideas for its proof. More than one hundred and fifty such proofs - more or less strict, more or less visual - are known, but the desire to increase their number has remained. I think that independent “discovery” of proofs of the Pythagorean theorem will be useful for modern schoolchildren.

Let's look at some examples of evidence that can suggest the direction of such searches.

Pythagorean proof

"A square built on the hypotenuse of a right triangle is equal to the sum of the squares built on its legs." The simplest proof of the theorem is obtained in the simplest case of an isosceles right triangle. This is probably where the theorem began. In fact, it is enough just to look at the mosaic of isosceles right triangles to be convinced of the validity of the theorem. For example, for DABC: a square built on the hypotenuse AC, contains 4 original triangles, and squares built on legs of two. The theorem has been proven.

Proofs based on the use of the concept of equal size of figures.

In this case, we can consider evidence in which a square built on the hypotenuse of a given right triangle is “composed” of the same figures as squares built on the sides. We can also consider proofs that use rearrangements of the summands of the figures and take into account a number of new ideas.

In Fig. 2 shows two equal square. The length of the sides of each square is a + b. Each of the squares is divided into parts consisting of squares and right triangles. It is clear that if we subtract quadruple the area of ​​a right triangle with legs a, b from the area of ​​the square, then we will be left with equal areas, i.e. c 2 = a 2 + b 2 . However, the ancient Hindus, to whom this reasoning belongs, usually did not write it down, but accompanied the drawing with only one word: “look!” It is quite possible that Pythagoras offered the same proof.

Additive evidence.

These proofs are based on the decomposition of squares built on the legs into figures from which one can add a square built on the hypotenuse.

Here: ABC is a right triangle with right angle C; CMN; CKMN; PO||MN; EF||MN.

Independently prove the pairwise equality of triangles obtained by partitioning squares built on the legs and hypotenuse.

Prove the theorem using this partition.

 Based on the proof of al-Nayriziyah, another decomposition of squares into pairs was carried out equal figures(Fig. 5, here ABC is a right triangle with right angle C).

 Another proof by the method of decomposing squares into equal parts, called the “wheel with blades,” is shown in Fig. 6. Here: ABC is a right triangle with right angle C; O is the center of a square built on a large side; dotted lines passing through point O are perpendicular or parallel to the hypotenuse.

 This decomposition of squares is interesting because it is pairwise equal quadrilaterals can be mapped onto each other parallel transfer. Many other proofs of the Pythagorean theorem can be offered using the decomposition of squares into figures.

Evidence by the method of completion.

The essence of this method is that equal figures are added to the squares built on the legs and to the square built on the hypotenuse in such a way that equal figures are obtained.

The validity of the Pythagorean theorem follows from the equal size of the hexagons AEDFPB and ACBNMQ. Here CEP, line EP divides the hexagon AEDFPB into two equal quadrilaterals, line CM divides the hexagon ACBNMQ into two equal quadrilaterals; Rotating the plane 90° around center A maps the quadrilateral AEPB onto the quadrilateral ACMQ.

In Fig. 8 The Pythagorean figure is completed to a rectangle, the sides of which are parallel to the corresponding sides of the squares built on the sides. Let's divide this rectangle into triangles and rectangles. From the resulting rectangle, we first subtract all the polygons 1, 2, 3, 4, 5, 6, 7, 8, 9, leaving a square built on the hypotenuse. Then from the same rectangle we subtract rectangles 5, 6, 7 and the shaded rectangles, we get squares built on the legs.

Now let us prove that the figures subtracted in the first case are equal in size to the figures subtracted in the second case.

KLOA = ACPF = ACED = a 2 ;

LGBO = CBMP = CBNQ = b 2 ;

AKGB = AKLO + LGBO = c 2 ;

hence c 2 = a 2 + b 2 .

OCLP = ACLF = ACED = b 2 ;

CBML = CBNQ = a 2 ;

OBMP = ABMF = c 2 ;

OBMP = OCLP + CBML;

c 2 = a 2 + b 2 .

Algebraic method of proof.

	Rice. 12 illustrates the proof of the great Indian mathematician Bhaskari (famous author Lilavati, X II century). The drawing was accompanied by only one word: LOOK! Among the proofs of the Pythagorean theorem algebraic method The first place (perhaps the oldest) is occupied by the proof using similarity.
	Let's bring in modern presentation one such proof belongs to Pythagoras.

N and fig. 13 ABC – rectangular, C – right angle, CMAB, b 1 – projection of leg b onto the hypotenuse, a 1 – projection of leg a onto the hypotenuse, h – altitude of the triangle drawn to the hypotenuse.

From the fact that ABC is similar to ACM it follows

b 2 = cb 1 ; (1)

from the fact that ABC is similar to BCM it follows

a 2 = ca 1 . (2)

Adding equalities (1) and (2) term by term, we obtain a 2 + b 2 = cb 1 + ca 1 = c(b 1 + a 1) = c 2 .

If Pythagoras really proposed such a proof, then he was also familiar with a number of important geometric theorems that modern historians mathematicians usually attribute it to Euclid.

Moehlmann's proof (Fig. 14). The area of ​​a given right triangle, on the one hand, is equal to the other, where p is the semi-perimeter of the triangle, r is the radius of the circle inscribed in it We have:

whence it follows that c 2 =a 2 +b 2.

in the second

Equating these expressions, we obtain the Pythagorean theorem.

Combined method

Equality of triangles

c 2 = a 2 + b 2 . (3)

Comparing relations (3) and (4), we obtain that

c 1 2 = c 2, or c 1 = c.

Thus, the triangles - given and constructed - are equal, since they have three respectively equal sides. Angle C 1 is right, therefore angle C given triangle also straight.

Ancient Indian evidence.

Mathematics Ancient India noticed that to prove the Pythagorean theorem it is enough to use the internal part of an ancient Chinese drawing. In the treatise “Siddhanta Shiromani” (“Crown of Knowledge”) written on palm leaves by the greatest Indian mathematician of the 19th century. Bha-skaras are placed in a drawing (Fig. 4)

characteristic of Indian evidence is the word “look!” As you can see, right triangles are laid here with the hypotenuse facing outwards and a square With 2 transferred to the “bride’s chair” With 2 -b 2 . Note that special cases of the Pythagorean theorem (for example, constructing a square whose area is twice as large Fig.4 area given square) found in the ancient Indian treatise "Sulva"

We solved a right triangle and squares built on its legs, or, in other words, figures made up of 16 identical isosceles right triangles and therefore fitting into a square. That's how lily is. a small fraction of the wealth hidden in the pearl of ancient mathematics - the Pythagorean theorem.

Ancient Chinese evidence.

Mathematical treatises of Ancient China have come down to us in the edition of the 2nd century. BC. The fact is that in 213 BC. Chinese Emperor Shi Huang Di, trying to eliminate previous traditions, ordered all ancient books to be burned. In P century BC. In China, paper was invented and at the same time the reconstruction of ancient books began. The most important of the surviving astronomical works is the book “Mathematics” containing a drawing (Fig. 2, a) proving the Pythagorean theorem. The key to this proof is not difficult to find. In fact, in the ancient Chinese drawing there are four equal right-angled triangles with sides a, b and the hypotenuse With stacked G) so that their outer contour forms Fig. 2 a square with side a+b, and the inner one is a square with side c, built on the hypotenuse (Fig. 2, b). If a square with side c is cut out and the remaining 4 shaded triangles are placed in two rectangles (Fig. 2, V), then it is clear that the resulting void, on the one hand, is equal to WITH 2 , and on the other - With 2 +b 2 , those. c 2=  2 +b 2 . The theorem has been proven. Note that with this proof, the constructions inside the square on the hypotenuse, which we see in the ancient Chinese drawing (Fig. 2, a), are not used. Apparently, ancient Chinese mathematicians had a different proof. Precisely if in a square with side With two shaded triangles (Fig. 2, b) cut off and attach the hypotenuses to the other two hypotenuses (Fig. 2, G), then it is easy to discover that

The resulting figure, sometimes called the "bride's chair", consists of two squares with sides A And b, those. c 2 == a 2 +b 2 .

N and Figure 3 reproduces a drawing from the treatise “Zhou-bi...”. Here the Pythagorean theorem is considered for Egyptian triangle with legs 3, 4 and hypotenuse 5 units of measurement. The square on the hypotenuse contains 25 cells, and the square inscribed in it on the larger leg contains 16. It is clear that the remaining part contains 9 cells. This will be the square on the smaller side.