Maintaining your privacy is important to us. For this reason, we have developed a Privacy Policy that describes how we use and store your information. Please review our privacy practices and let us know if you have any questions.

Collection and use of personal information

Personal information refers to data that can be used to identify or contact a specific person.

You may be asked to provide your personal information at any time when you contact us.

Below are some examples of the types of personal information we may collect and how we may use such information.

What personal information do we collect:

• When you submit an application on the site, we may collect various information, including your name, telephone number, email address, etc.

How we use your personal information:

• The personal information we collect allows us to contact you with unique offers, promotions and other events and upcoming events.
• From time to time, we may use your personal information to send important notices and communications.
• We may also use personal information for internal purposes, such as conducting audits, data analysis and various research in order to improve the services we provide and provide you with recommendations regarding our services.
• If you participate in a prize draw, contest or similar promotion, we may use the information you provide to administer such programs.

Disclosure of information to third parties

We do not disclose the information received from you to third parties.

Exceptions:

• If necessary - in accordance with the law, judicial procedure, in legal proceedings, and/or on the basis of public requests or requests from government authorities in the territory of the Russian Federation - to disclose your personal information. We may also disclose information about you if we determine that such disclosure is necessary or appropriate for security, law enforcement, or other public importance purposes.
• In the event of a reorganization, merger, or sale, we may transfer the personal information we collect to the applicable successor third party.

Protection of personal information

We take precautions - including administrative, technical and physical - to protect your personal information from loss, theft, and misuse, as well as unauthorized access, disclosure, alteration and destruction.

Respecting your privacy at the company level

To ensure that your personal information is secure, we communicate privacy and security standards to our employees and strictly enforce privacy practices.

Lesson content

Linear equations in two variables

A schoolchild has 200 rubles to eat lunch at school. A cake costs 25 rubles, and a cup of coffee costs 10 rubles. How many cakes and cups of coffee can you buy for 200 rubles?

Let us denote the number of cakes by x, and the number of cups of coffee through y. Then the cost of the cakes will be denoted by the expression 25 x, and the cost of cups of coffee in 10 y .

25x— price x cakes
10y — price y cups of coffee

The total amount should be 200 rubles. Then we get an equation with two variables x And y

25x+ 10y= 200

How many roots does this equation have?

It all depends on the student’s appetite. If he buys 6 cakes and 5 cups of coffee, then the roots of the equation will be the numbers 6 and 5.

The pair of values ​​6 and 5 are said to be the roots of equation 25 x+ 10y= 200 . Written as (6; 5), with the first number being the value of the variable x, and the second - the value of the variable y .

6 and 5 are not the only roots that reverse equation 25 x+ 10y= 200 to identity. If desired, for the same 200 rubles a student can buy 4 cakes and 10 cups of coffee:

In this case, the roots of equation 25 x+ 10y= 200 is a pair of values ​​(4; 10).

Moreover, a schoolchild may not buy coffee at all, but buy cakes for the entire 200 rubles. Then the roots of equation 25 x+ 10y= 200 will be the values ​​8 and 0

Or vice versa, don’t buy cakes, but buy coffee for the entire 200 rubles. Then the roots of equation 25 x+ 10y= 200 the values ​​will be 0 and 20

Let's try to list all possible roots of equation 25 x+ 10y= 200 . Let us agree that the values x And y belong to the set of integers. And let these values ​​be greater than or equal to zero:

x∈Z, y∈ Z;
x ≥ 0, y ≥ 0

This will be convenient for the student himself. It is more convenient to buy whole cakes than, for example, several whole cakes and half a cake. It is also more convenient to take coffee in whole cups than, for example, several whole cups and half a cup.

Note that for odd x it is impossible to achieve equality under any circumstances y. Then the values x the following numbers will be 0, 2, 4, 6, 8. And knowing x can be easily determined y

Thus, we received the following pairs of values (0; 20), (2; 15), (4; 10), (6; 5), (8; 0). These pairs are solutions or roots of Equation 25 x+ 10y= 200. They turn this equation into an identity.

Equation of the form ax + by = c called linear equation with two variables. The solution or roots of this equation are a pair of values ​​( x; y), which turns it into identity.

Note also that if a linear equation with two variables is written in the form ax + b y = c , then they say that it is written in canonical(normal) form.

Some linear equations in two variables can be reduced to canonical form.

For example, the equation 2(16x+ 3y − 4) = 2(12 + 8x − y) can be brought to mind ax + by = c. Let's open the brackets on both sides of this equation and get 32x + 6y − 8 = 24 + 16x − 2y . We group terms containing unknowns on the left side of the equation, and terms free of unknowns - on the right. Then we get 32x− 16x+ 6y+ 2y = 24 + 8 . We present similar terms in both sides, we get equation 16 x+ 8y= 32. This equation is reduced to the form ax + by = c and is canonical.

Equation 25 discussed earlier x+ 10y= 200 is also a linear equation with two variables in canonical form. In this equation the parameters a , b And c are equal to the values ​​25, 10 and 200, respectively.

Actually the equation ax + by = c has countless solutions. Solving the equation 25x+ 10y= 200, we looked for its roots only on the set of integers. As a result, we obtained several pairs of values ​​that turned this equation into an identity. But on the set of rational numbers, equation 25 x+ 10y= 200 will have infinitely many solutions.

To obtain new pairs of values, you need to take an arbitrary value for x, then express y. For example, let's take for the variable x value 7. Then we get an equation with one variable 25×7 + 10y= 200 in which one can express y

Let x= 15. Then the equation 25x+ 10y= 200 becomes 25 × 15 + 10y= 200. From here we find that y = −17,5

Let x= −3 . Then the equation 25x+ 10y= 200 becomes 25 × (−3) + 10y= 200. From here we find that y = −27,5

System of two linear equations with two variables

For the equation ax + by = c you can take arbitrary values ​​for as many times as you like x and find values ​​for y. Taken separately, such an equation will have countless solutions.

But it also happens that the variables x And y connected not by one, but by two equations. In this case they form the so-called system of linear equations in two variables. Such a system of equations can have one pair of values ​​(or in other words: “one solution”).

It may also happen that the system has no solutions at all. A system of linear equations can have countless solutions in rare and exceptional cases.

Two linear equations form a system when the values x And y enter into each of these equations.

Let's go back to the very first equation 25 x+ 10y= 200 . One of the pairs of values ​​for this equation was the pair (6; 5) . This is a case when for 200 rubles you could buy 6 cakes and 5 cups of coffee.

Let's formulate the problem so that the pair (6; 5) becomes the only solution for equation 25 x+ 10y= 200 . To do this, let’s create another equation that would connect the same x cakes and y cups of coffee.

Let us state the text of the problem as follows:

“The student bought several cakes and several cups of coffee for 200 rubles. A cake costs 25 rubles, and a cup of coffee costs 10 rubles. How many cakes and cups of coffee did the student buy if it is known that the number of cakes is one unit greater than the number of cups of coffee?

We already have the first equation. This is equation 25 x+ 10y= 200 . Now let's create an equation for the condition “the number of cakes is one unit greater than the number of cups of coffee” .

The number of cakes is x, and the number of cups of coffee is y. You can write this phrase using the equation x−y= 1. This equation will mean that the difference between cakes and coffee is 1.

x = y+ 1 . This equation means that the number of cakes is one more than the number of cups of coffee. Therefore, to obtain equality, one is added to the number of cups of coffee. This can be easily understood if we use the model of scales that we considered when studying the simplest problems:

We got two equations: 25 x+ 10y= 200 and x = y+ 1. Since the values x And y, namely 6 and 5 are included in each of these equations, then together they form a system. Let's write down this system. If the equations form a system, then they are framed by the system sign. The system symbol is a curly brace:

Let's solve this system. This will allow us to see how we arrive at the values ​​6 and 5. There are many methods for solving such systems. Let's look at the most popular of them.

Substitution method

The name of this method speaks for itself. Its essence is to substitute one equation into another, having previously expressed one of the variables.

In our system, nothing needs to be expressed. In the second equation x = y+ 1 variable x already expressed. This variable is equal to the expression y+ 1 . Then you can substitute this expression into the first equation instead of the variable x

After substituting the expression y+ 1 into the first equation instead x, we get the equation 25(y+ 1) + 10y= 200 . This is a linear equation with one variable. This equation is quite easy to solve:

We found the value of the variable y. Now let's substitute this value into one of the equations and find the value x. For this it is convenient to use the second equation x = y+ 1 . Let’s substitute the value into it y

This means that the pair (6; 5) is a solution to the system of equations, as we intended. We check and make sure that the pair (6; 5) satisfies the system:

Example 2

Let's substitute the first equation x= 2 + y into the second equation 3 x− 2y= 9. In the first equation the variable x equal to the expression 2 + y. Let’s substitute this expression into the second equation instead of x

Now let's find the value x. To do this, let's substitute the value y into the first equation x= 2 + y

This means that the solution to the system is the pair value (5; 3)

Example 3. Solve the following system of equations using the substitution method:

Here, unlike previous examples, one of the variables is not expressed explicitly.

To substitute one equation into another, you first need .

It is advisable to express the variable that has a coefficient of one. The variable has a coefficient of one x, which is contained in the first equation x+ 2y= 11. Let's express this variable.

After variable expression x, our system will take the following form:

Now let's substitute the first equation into the second and find the value y

Let's substitute y x

This means that the solution to the system is a pair of values ​​(3; 4)

Of course, you can also express a variable y. This will not change the roots. But if you express y, The result is not a very simple equation, which will take more time to solve. It will look like this:

We see that in this example we express x much more convenient than expressing y .

Example 4. Solve the following system of equations using the substitution method:

Let us express in the first equation x. Then the system will take the form:

y

Let's substitute y into the first equation and find x. You can use the original equation 7 x+ 9y= 8, or use the equation in which the variable is expressed x. We will use this equation because it is convenient:

This means that the solution to the system is a pair of values ​​(5; −3)

Addition method

The addition method consists of adding the equations included in the system term by term. This addition results in a new equation with one variable. And solving such an equation is quite simple.

Let's solve the following system of equations:

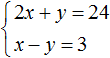
Let's add the left side of the first equation with the left side of the second equation. And the right side of the first equation with the right side of the second equation. We get the following equality:

Let's look at similar terms:

As a result, we got the simplest equation 3 x= 27 whose root is 9. Knowing the value x you can find the value y. Let's substitute the value x into the second equation x−y= 3 . We get 9 − y= 3 . From here y= 6 .

This means that the solution to the system is a pair of values ​​(9; 6)

Example 2

Let's add the left side of the first equation with the left side of the second equation. And the right side of the first equation with the right side of the second equation. In the resulting equality we present similar terms:

As a result, we got the simplest equation 5 x= 20, whose root is 4. Knowing the value x you can find the value y. Let's substitute the value x into the first equation 2 x+y= 11. Let's get 8+ y= 11. From here y= 3 .

This means that the solution to the system is a pair of values ​​(4;3)

The addition process is not described in detail. It must be done mentally. When adding, both equations must be reduced to canonical form. That is, by the way ac + by = c .

From the examples considered, it is clear that the main purpose of adding equations is to get rid of one of the variables. But it is not always possible to immediately solve a system of equations using the addition method. Most often, the system is first brought to a form in which the equations included in this system can be added.

For example, the system can be solved immediately by addition. When adding both equations, the terms y And −y will disappear because their sum is zero. As a result, the simplest equation 11 is formed x= 22, whose root is 2. It will then be possible to determine y equal to 5.

And the system of equations The addition method cannot be solved immediately, since this will not lead to the disappearance of one of the variables. Addition will result in equation 8 x+ y= 28, which has an infinite number of solutions.

If both sides of the equation are multiplied or divided by the same number, not equal to zero, you get an equation equivalent to the given one. This rule is also true for a system of linear equations with two variables. One of the equations (or both equations) can be multiplied by any number. The result will be an equivalent system, the roots of which will coincide with the previous one.

Let's return to the very first system, which described how many cakes and cups of coffee a schoolchild bought. The solution to this system was a pair of values ​​(6; 5).

Let's multiply both equations included in this system by some numbers. Let's say we multiply the first equation by 2, and the second by 3

As a result, we got a system
The solution to this system is still the pair of values ​​(6; 5)

This means that the equations included in the system can be reduced to a form suitable for applying the addition method.

Let's return to the system , which we could not solve using the addition method.

Multiply the first equation by 6, and the second by −2

Then we get the following system:

Let's add up the equations included in this system. Adding components 12 x and −12 x will result in 0, addition 18 y and 4 y will give 22 y, and adding 108 and −20 gives 88. Then we get equation 22 y= 88, from here y = 4 .

If at first it’s hard to add equations in your head, then you can write down how the left side of the first equation adds up with the left side of the second equation, and the right side of the first equation with the right side of the second equation:

Knowing that the value of the variable y equals 4, you can find the value x. Let's substitute y into one of the equations, for example into the first equation 2 x+ 3y= 18. Then we get an equation with one variable 2 x+ 12 = 18. Let's move 12 to the right side, changing the sign, we get 2 x= 6, from here x = 3 .

Example 4. Solve the following system of equations using the addition method:

Let's multiply the second equation by −1. Then the system will take the following form:

Let's add both equations. Adding components x And −x will result in 0, addition 5 y and 3 y will give 8 y, and adding 7 and 1 gives 8. The result is equation 8 y= 8 whose root is 1. Knowing that the value y equals 1, you can find the value x .

Let's substitute y into the first equation, we get x+ 5 = 7, hence x= 2

Example 5. Solve the following system of equations using the addition method:

It is desirable that terms containing the same variables be located one below the other. Therefore, in the second equation the terms 5 y and −2 x Let's swap places. As a result, the system will take the form:

Let's multiply the second equation by 3. Then the system will take the form:

Now let's add both equations. As a result of addition we obtain equation 8 y= 16, whose root is 2.

Let's substitute y into the first equation, we get 6 x− 14 = 40. Let's move the term −14 to the right side, changing the sign, and get 6 x= 54 . From here x= 9.

Example 6. Solve the following system of equations using the addition method:

Let's get rid of fractions. Multiply the first equation by 36, and the second by 12

In the resulting system the first equation can be multiplied by −5, and the second by 8

Let's add up the equations in the resulting system. Then we get the simplest equation −13 y= −156 . From here y= 12. Let's substitute y into the first equation and find x

Example 7. Solve the following system of equations using the addition method:

Let us bring both equations to normal form. Here it is convenient to apply the rule of proportion in both equations. If in the first equation the right side is represented as , and the right side of the second equation as , then the system will take the form:

We have a proportion. Let's multiply its extreme and middle terms. Then the system will take the form:

Let's multiply the first equation by −3, and open the brackets in the second:

Now let's add both equations. As a result of adding these equations, we get an equality with zero on both sides:

It turns out that the system has countless solutions.

But we can’t just take arbitrary values ​​from the sky for x And y. We can specify one of the values, and the other will be determined depending on the value we specify. For example, let x= 2 . Let's substitute this value into the system:

As a result of solving one of the equations, the value for y, which will satisfy both equations:

The resulting pair of values ​​(2; −2) will satisfy the system:

Let's find another pair of values. Let x= 4. Let's substitute this value into the system:

You can tell by eye that the value y equals zero. Then we get a pair of values ​​(4; 0) that satisfies our system:

Example 8. Solve the following system of equations using the addition method:

Multiply the first equation by 6 and the second by 12

Let's rewrite what's left:

Let's multiply the first equation by −1. Then the system will take the form:

Now let's add both equations. As a result of addition, equation 6 is formed b= 48, whose root is 8. Substitute b into the first equation and find a

System of linear equations with three variables

A linear equation with three variables includes three variables with coefficients, as well as an intercept term. In canonical form it can be written as follows:

ax + by + cz = d

This equation has countless solutions. By giving two variables different values, a third value can be found. The solution in this case is a triple of values ​​( x; y; z) which turns the equation into an identity.

If the variables x, y, z are interconnected by three equations, then a system of three linear equations with three variables is formed. To solve such a system, you can use the same methods that apply to linear equations with two variables: the substitution method and the addition method.

Example 1. Solve the following system of equations using the substitution method:

Let us express in the third equation x. Then the system will take the form:

Now let's do the substitution. Variable x is equal to the expression 3 − 2y − 2z . Let's substitute this expression into the first and second equations:

Let's open the brackets in both equations and present similar terms:

We have arrived at a system of linear equations with two variables. In this case, it is convenient to use the addition method. As a result, the variable y will disappear and we can find the value of the variable z

Now let's find the value y. To do this, it is convenient to use the equation − y+ z= 4. Substitute the value into it z

Now let's find the value x. To do this, it is convenient to use the equation x= 3 − 2y − 2z . Let's substitute the values ​​into it y And z

Thus, the triple of values ​​(3; −2; 2) is a solution to our system. By checking we make sure that these values ​​satisfy the system:

Example 2. Solve the system using the addition method

Let's add the first equation with the second, multiplied by −2.

If the second equation is multiplied by −2, it takes the form −6x+ 6y − 4z = −4 . Now let's add it to the first equation:

We see that as a result of elementary transformations, the value of the variable was determined x. It is equal to one.

Let's return to the main system. Let's add the second equation with the third, multiplied by −1. If the third equation is multiplied by −1, it takes the form −4x + 5y − 2z = −1 . Now let's add it to the second equation:

We got the equation x− 2y= −1 . Let's substitute the value into it x which we found earlier. Then we can determine the value y

Now we know the meanings x And y. This allows you to determine the value z. Let's use one of the equations included in the system:

Thus, the triple of values ​​(1; 1; 1) is the solution to our system. By checking we make sure that these values ​​satisfy the system:

Problems on composing systems of linear equations

The task of composing systems of equations is solved by entering several variables. Next, equations are compiled based on the conditions of the problem. From the compiled equations they form a system and solve it. Having solved the system, it is necessary to check whether its solution satisfies the conditions of the problem.

Problem 1. A Volga car drove out of the city to the collective farm. She returned back along another road, which was 5 km shorter than the first. In total, the car traveled 35 km round trip. How many kilometers is the length of each road?

Solution

Let x— length of the first road, y- length of the second. If the car traveled 35 km round trip, then the first equation can be written as x+ y= 35. This equation describes the sum of the lengths of both roads.

It is said that the car returned along a road that was 5 km shorter than the first one. Then the second equation can be written as x− y= 5. This equation shows that the difference between the road lengths is 5 km.

Or the second equation can be written as x= y+ 5. We will use this equation.

Because the variables x And y in both equations denote the same number, then we can form a system from them:

Let's solve this system using some of the previously studied methods. In this case, it is convenient to use the substitution method, since in the second equation the variable x already expressed.

Substitute the second equation into the first and find y

Let's substitute the found value y in the second equation x= y+ 5 and we'll find x

The length of the first road was designated through the variable x. Now we have found its meaning. Variable x is equal to 20. This means that the length of the first road is 20 km.

And the length of the second road was indicated by y. The value of this variable is 15. This means the length of the second road is 15 km.

Let's check. First, let's make sure that the system is solved correctly:

Now let’s check whether the solution (20; 15) satisfies the conditions of the problem.

It was said that the car traveled a total of 35 km round trip. We add the lengths of both roads and make sure that the solution (20; 15) satisfies this condition: 20 km + 15 km = 35 km

The following condition: the car returned back along another road, which was 5 km shorter than the first . We see that solution (20; 15) also satisfies this condition, since 15 km is shorter than 20 km by 5 km: 20 km − 15 km = 5 km

When composing a system, it is important that the variables represent the same numbers in all equations included in this system.

So our system contains two equations. These equations in turn contain variables x And y, which represent the same numbers in both equations, namely road lengths of 20 km and 15 km.

Problem 2. Oak and pine sleepers were loaded onto the platform, 300 sleepers in total. It is known that all oak sleepers weighed 1 ton less than all pine sleepers. Determine how many oak and pine sleepers there were separately, if each oak sleeper weighed 46 kg, and each pine sleeper 28 kg.

Solution

Let x oak and y pine sleepers were loaded onto the platform. If there were 300 sleepers in total, then the first equation can be written as x+y = 300 .

All oak sleepers weighed 46 x kg, and the pine ones weighed 28 y kg. Since oak sleepers weighed 1 ton less than pine sleepers, the second equation can be written as 28y − 46x= 1000 . This equation shows that the difference in mass between oak and pine sleepers is 1000 kg.

Tons were converted to kilograms since the mass of oak and pine sleepers was measured in kilograms.

As a result, we obtain two equations that form the system

Let's solve this system. Let us express in the first equation x. Then the system will take the form:

Substitute the first equation into the second and find y

Let's substitute y into the equation x= 300 − y and find out what it is x

This means that 100 oak and 200 pine sleepers were loaded onto the platform.

Let's check whether the solution (100; 200) satisfies the conditions of the problem. First, let's make sure that the system is solved correctly:

It was said that there were 300 sleepers in total. We add up the number of oak and pine sleepers and make sure that the solution (100; 200) satisfies this condition: 100 + 200 = 300.

The following condition: all oak sleepers weighed 1 ton less than all pine sleepers . We see that the solution (100; 200) also satisfies this condition, since 46 × 100 kg of oak sleepers is lighter than 28 × 200 kg of pine sleepers: 5600 kg − 4600 kg = 1000 kg.

Problem 3. We took three pieces of copper-nickel alloy in ratios of 2: 1, 3: 1 and 5: 1 by weight. A piece weighing 12 kg was fused from them with a ratio of copper and nickel content of 4: 1. Find the mass of each original piece if the mass of the first is twice the mass of the second.

To study a system of linear agebraic equations (SLAEs) for consistency means to find out whether this system has solutions or does not have them. Well, if there are solutions, then indicate how many there are.

We will need information from the topic "System of linear algebraic equations. Basic terms. Matrix form of notation". In particular, concepts such as system matrix and extended system matrix are needed, since the formulation of the Kronecker-Capelli theorem is based on them. As usual, we will denote the system matrix by the letter $A$, and the extended matrix of the system by the letter $\widetilde(A)$.

Kronecker-Capelli theorem

A system of linear algebraic equations is consistent if and only if the rank of the system matrix is ​​equal to the rank of the extended matrix of the system, i.e. $\rang A=\rang\widetilde(A)$.

Let me remind you that a system is called joint if it has at least one solution. The Kronecker-Capelli theorem says this: if $\rang A=\rang\widetilde(A)$, then there is a solution; if $\rang A\neq\rang\widetilde(A)$, then this SLAE has no solutions (inconsistent). The answer to the question about the number of these solutions is given by a corollary of the Kronecker-Capelli theorem. In the formulation of the corollary, the letter $n$ is used, which is equal to the number of variables of the given SLAE.

Corollary to the Kronecker-Capelli theorem

1. If $\rang A\neq\rang\widetilde(A)$, then the SLAE is inconsistent (has no solutions).
2. If $\rang A=\rang\widetilde(A)< n$, то СЛАУ является неопределённой (имеет бесконечное количество решений).
3. If $\rang A=\rang\widetilde(A) = n$, then the SLAE is definite (has exactly one solution).

Please note that the formulated theorem and its corollary do not indicate how to find a solution to the SLAE. With their help, you can only find out whether these solutions exist or not, and if they exist, then how many.

Example No. 1

Explore SLAE $ \left \(\begin(aligned) & -3x_1+9x_2-7x_3=17;\\ & -x_1+2x_2-4x_3=9;\\ & 4x_1-2x_2+19x_3=-42. \end(aligned )\right.$ for compatibility. If the SLAE is compatible, indicate the number of solutions.

To find out the existence of solutions to a given SLAE, we use the Kronecker-Capelli theorem. We will need the matrix of the system $A$ and the extended matrix of the system $\widetilde(A)$, we will write them:

$$ A=\left(\begin(array) (ccc) -3 & 9 & -7 \\ -1 & 2 & -4 \\ 4 & -2 & 19 \end(array) \right);\; \widetilde(A)=\left(\begin(array) (ccc|c) -3 & 9 &-7 & 17 \\ -1 & 2 & -4 & 9\\ 4 & -2 & 19 & -42 \end(array) \right). $$

We need to find $\rang A$ and $\rang\widetilde(A)$. There are many ways to do this, some of which are listed in the Matrix Rank section. Typically, two methods are used to study such systems: “Calculating the rank of a matrix by definition” or “Calculating the rank of a matrix by the method of elementary transformations”.

Method number 1. Computing ranks by definition.

According to the definition, rank is the highest order of the minors of a matrix, among which there is at least one that is different from zero. Usually, the study begins with first-order minors, but here it is more convenient to immediately begin calculating the third-order minor of the matrix $A$. The third-order minor elements are located at the intersection of three rows and three columns of the matrix in question. Since the matrix $A$ contains only 3 rows and 3 columns, the third order minor of the matrix $A$ is the determinant of the matrix $A$, i.e. $\Delta A$. To calculate the determinant, we apply formula No. 2 from the topic “Formulas for calculating determinants of the second and third orders”:

$$ \Delta A=\left| \begin(array) (ccc) -3 & 9 & -7 \\ -1 & 2 & -4 \\ 4 & -2 & 19 \end(array) \right|=-21. $$

So, there is a third order minor of the matrix $A$, which is not equal to zero. It is impossible to construct a fourth-order minor, since it requires 4 rows and 4 columns, and the matrix $A$ has only 3 rows and 3 columns. So, the highest order of the minors of the matrix $A$, among which there is at least one that is not equal to zero, is equal to 3. Therefore, $\rang A=3$.

We also need to find $\rang\widetilde(A)$. Let's look at the structure of the matrix $\widetilde(A)$. Up to the line in the matrix $\widetilde(A)$ there are elements of the matrix $A$, and we found out that $\Delta A\neq 0$. Consequently, the matrix $\widetilde(A)$ has a third-order minor, which is not equal to zero. We cannot construct fourth-order minors of the matrix $\widetilde(A)$, so we conclude: $\rang\widetilde(A)=3$.

Since $\rang A=\rang\widetilde(A)$, then according to the Kronecker-Capelli theorem the system is consistent, i.e. has a solution (at least one). To indicate the number of solutions, we take into account that our SLAE contains 3 unknowns: $x_1$, $x_2$ and $x_3$. Since the number of unknowns is $n=3$, we conclude: $\rang A=\rang\widetilde(A)=n$, therefore, according to the corollary of the Kronecker-Capelli theorem, the system is definite, i.e. has a unique solution.

The problem is solved. What disadvantages and advantages does this method have? First, let's talk about the advantages. Firstly, we only needed to find one determinant. After this, we immediately made a conclusion about the number of solutions. Typically, standard standard calculations give systems of equations that contain three unknowns and have a unique solution. For such systems, this method is very convenient, because we know in advance that there is a solution (otherwise the example would not have been in the standard calculation). Those. All we have to do is show the existence of a solution in the fastest way. Secondly, the calculated value of the determinant of the system matrix (i.e. $\Delta A$) will be useful later: when we begin to solve a given system using the Cramer method or using the inverse matrix.

However, the method of calculating the rank is by definition undesirable to use if the matrix of the system $A$ is rectangular. In this case, it is better to use the second method, which will be discussed below. In addition, if $\Delta A=0$, then we cannot say anything about the number of solutions of a given inhomogeneous SLAE. Maybe the SLAE has an infinite number of solutions, or maybe none. If $\Delta A=0$, then additional research is required, which is often cumbersome.

To summarize what has been said, I note that the first method is good for those SLAEs whose system matrix is ​​square. Moreover, the SLAE itself contains three or four unknowns and is taken from standard standard calculations or tests.

Method number 2. Calculation of rank by the method of elementary transformations.

This method is described in detail in the corresponding topic. We will begin to calculate the rank of the matrix $\widetilde(A)$. Why matrices $\widetilde(A)$ and not $A$? The fact is that the matrix $A$ is part of the matrix $\widetilde(A)$, therefore, by calculating the rank of the matrix $\widetilde(A)$ we will simultaneously find the rank of the matrix $A$.

\begin(aligned) &\widetilde(A) =\left(\begin(array) (ccc|c) -3 & 9 &-7 & 17 \\ -1 & 2 & -4 & 9\\ 4 & - 2 & 19 & -42 \end(array) \right) \rightarrow \left|\text(swap the first and second lines)\right| \rightarrow \\ &\rightarrow \left(\begin(array) (ccc|c) -1 & 2 & -4 & 9 \\ -3 & 9 &-7 & 17\\ 4 & -2 & 19 & - 42 \end(array) \right) \begin(array) (l) \phantom(0) \\ II-3\cdot I\\ III+4\cdot I \end(array) \rightarrow \left(\begin (array) (ccc|c) -1 & 2 & -4 & 9 \\ 0 & 3 &5 & -10\\ 0 & 6 & 3 & -6 \end(array) \right) \begin(array) ( l) \phantom(0) \\ \phantom(0)\\ III-2\cdot II \end(array)\rightarrow\\ &\rightarrow \left(\begin(array) (ccc|c) -1 & 2 & -4 & 9 \\ 0 & 3 &5 & -10\\ 0 & 0 & -7 & 14 \end(array) \right) \end(aligned)

We have reduced the matrix $\widetilde(A)$ to trapezoidal form. On the main diagonal of the resulting matrix $\left(\begin(array) (ccc|c) -1 & 2 & -4 & 9 \\ 0 & 3 &5 & -10\\ 0 & 0 & -7 & 14 \end( array) \right)$ contains three non-zero elements: -1, 3 and -7. Conclusion: the rank of the matrix $\widetilde(A)$ is 3, i.e. $\rang\widetilde(A)=3$. When making transformations with the elements of the matrix $\widetilde(A)$, we simultaneously transformed the elements of the matrix $A$ located up to the line. The matrix $A$ is also reduced to trapezoidal form: $\left(\begin(array) (ccc) -1 & 2 & -4 \\ 0 & 3 &5 \\ 0 & 0 & -7 \end(array) \right )$. Conclusion: the rank of matrix $A$ is also 3, i.e. $\rang A=3$.

Since $\rang A=\rang\widetilde(A)$, then according to the Kronecker-Capelli theorem the system is consistent, i.e. has a solution. To indicate the number of solutions, we take into account that our SLAE contains 3 unknowns: $x_1$, $x_2$ and $x_3$. Since the number of unknowns is $n=3$, we conclude: $\rang A=\rang\widetilde(A)=n$, therefore, according to the corollary of the Kronecker-Capelli theorem, the system is defined, i.e. has a unique solution.

What are the advantages of the second method? The main advantage is its versatility. It doesn't matter to us whether the matrix of the system is square or not. In addition, we actually carried out forward transformations of the Gaussian method. There are only a couple of steps left, and we could obtain a solution to this SLAE. To be honest, I like the second method more than the first, but the choice is a matter of taste.

Answer: The given SLAE is consistent and defined.

Example No. 2

Explore SLAE $ \left\( \begin(aligned) & x_1-x_2+2x_3=-1;\\ & -x_1+2x_2-3x_3=3;\\ & 2x_1-x_2+3x_3=2;\\ & 3x_1- 2x_2+5x_3=1;\\ & 2x_1-3x_2+5x_3=-4.\end(aligned) \right.$ for compatibility.

We will find the ranks of the system matrix and the extended system matrix using the method of elementary transformations. Extended system matrix: $\widetilde(A)=\left(\begin(array) (ccc|c) 1 & -1 & 2 & -1\\ -1 & 2 & -3 & 3 \\ 2 & -1 & 3 & 2 \\ 3 & -2 & 5 & 1 \\ 2 & -3 & 5 & -4 \end(array) \right)$. Let's find the required ranks by transforming the extended matrix of the system:

The extended matrix of the system is reduced to a stepwise form. If a matrix is ​​reduced to echelon form, then its rank is equal to the number of non-zero rows. Therefore, $\rang A=3$. The matrix $A$ (up to the line) is reduced to trapezoidal form and its rank is 2, $\rang A=2$.

Since $\rang A\neq\rang\widetilde(A)$, then according to the Kronecker-Capelli theorem the system is inconsistent (i.e., has no solutions).

Answer: The system is inconsistent.

Example No. 3

Explore SLAE $ \left\( \begin(aligned) & 2x_1+7x_3-5x_4+11x_5=42;\\ & x_1-2x_2+3x_3+2x_5=17;\\ & -3x_1+9x_2-11x_3-7x_5=-64 ;\\ & -5x_1+17x_2-16x_3-5x_4-4x_5=-90;\\ & 7x_1-17x_2+23x_3+15x_5=132. \end(aligned) \right.$ for compatibility.

The extended matrix of the system has the form: $\widetilde(A)=\left(\begin(array) (ccccc|c) 2 & 0 & 7 & -5 & 11 & 42\\ 1 & -2 & 3 & 0 & 2 & 17 \\ -3 & 9 & -11 & 0 & -7 & -64 \\ -5 & 17 & -16 & -5 & -4 & -90 \\ 7 & -17 & 23 & 0 & 15 & 132 \end(array) \right)$. Let's swap the first and second rows of this matrix so that the first element of the first row becomes one: $\left(\begin(array) (ccccc|c) 1 & -2 & 3 & 0 & 2 & 17\\ 2 & 0 & 7 & -5 & 11 & 42 \\ -3 & 9 & -11 & 0 & -7 & -64 \\ -5 & 17 & -16 & -5 & -4 & -90 \\ 7 & -17 & 23 & 0 & 15 & 132 \end(array) \right)$.

We have reduced the extended matrix of the system and the matrix of the system itself to a trapezoidal form. The rank of the extended matrix of the system is equal to three, the rank of the matrix of the system is also equal to three. Since the system contains $n=5$ unknowns, i.e. $\rang\widetilde(A)=\rang A< n$, то согласно следствия из теоремы Кронекера-Капелли данная система является неопределённой, т.е. имеет бесконечное количество решений.

Answer: The system is uncertain.

In the second part, we will analyze examples that are often included in standard calculations or tests in higher mathematics: consistency research and solution of SLAE depending on the values ​​of the parameters included in it.

In this lesson we will look at methods for solving a system of linear equations. In a course of higher mathematics, systems of linear equations are required to be solved both in the form of separate tasks, for example, “Solve the system using Cramer’s formulas,” and in the course of solving other problems. Systems of linear equations have to be dealt with in almost all branches of higher mathematics.

First, a little theory. What does the mathematical word “linear” mean in this case? This means that the equations of the system All variables included in the first degree: without any fancy stuff like etc., which only participants in mathematical Olympiads are delighted with.

In higher mathematics, not only letters familiar from childhood are used to denote variables.
A fairly popular option is variables with indexes: .
Or the initial letters of the Latin alphabet, small and large:
It is not so rare to find Greek letters: – known to many as “alpha, beta, gamma”. And also a set with indices, say, with the letter “mu”:

The use of one or another set of letters depends on the section of higher mathematics in which we are faced with a system of linear equations. So, for example, in systems of linear equations encountered when solving integrals and differential equations, it is traditional to use the notation

But no matter how the variables are designated, the principles, methods and methods for solving a system of linear equations do not change. Thus, if you come across something scary like , do not rush to close the problem book in fear, after all, you can draw the sun instead, a bird instead, and a face (the teacher) instead. And, funny as it may seem, a system of linear equations with these notations can also be solved.

I have a feeling that the article will turn out to be quite long, so a small table of contents. So, the sequential “debriefing” will be like this:

– Solving a system of linear equations using the substitution method (“school method”);
– Solving the system by term-by-term addition (subtraction) of the system equations;
– Solution of the system using Cramer’s formulas;
– Solving the system using an inverse matrix;
– Solving the system using the Gaussian method.

Everyone is familiar with systems of linear equations from school mathematics courses. Essentially, we start with repetition.

Solving a system of linear equations using the substitution method

This method can also be called the “school method” or the method of eliminating unknowns. Figuratively speaking, it can also be called “an unfinished Gaussian method.”

Example 1

Here we are given a system of two equations with two unknowns. Note that the free terms (numbers 5 and 7) are located on the left side of the equation. Generally speaking, it doesn’t matter where they are, on the left or on the right, it’s just that in problems in higher mathematics they are often located that way. And such a recording should not lead to confusion; if necessary, the system can always be written “as usual”: . Don’t forget that when moving a term from part to part, it needs to change its sign.

What does it mean to solve a system of linear equations? Solving a system of equations means finding many of its solutions. The solution of a system is a set of values ​​of all variables included in it, which turns EVERY equation of the system into a true equality. In addition, the system can be non-joint (have no solutions).Don’t be shy, this is a general definition =) We will have only one “x” value and one “y” value, which satisfy each c-we equation.

There is a graphical method for solving the system, which you can familiarize yourself with in class. The simplest problems with a line. There I talked about geometric sense systems of two linear equations with two unknowns. But now this is the era of algebra, and numbers-numbers, actions-actions.

Let's decide: from the first equation we express:
We substitute the resulting expression into the second equation:

We open the brackets, add similar terms and find the value:

Next, we remember what we danced for:
We already know the value, all that remains is to find:

Answer:

After ANY system of equations has been solved in ANY way, I strongly recommend checking (orally, on a draft or on a calculator). Fortunately, this is done easily and quickly.

1) Substitute the found answer into the first equation:

– the correct equality is obtained.

2) Substitute the found answer into the second equation:

– the correct equality is obtained.

Or, to put it more simply, “everything came together”

The considered method of solution is not the only one; from the first equation it was possible to express , and not .
You can do the opposite - express something from the second equation and substitute it into the first equation. By the way, note that the most disadvantageous of the four methods is to express from the second equation:

The result is fractions, but why? There is a more rational solution.

However, in some cases you still can’t do without fractions. In this regard, I would like to draw your attention to HOW I wrote down the expression. Not like this: and in no case like this: .

If in higher mathematics you are dealing with fractional numbers, then try to carry out all calculations in ordinary improper fractions.

Exactly, and not or!

A comma can be used only sometimes, in particular if it is the final answer to some problem, and no further actions need to be performed with this number.

Many readers probably thought “why such a detailed explanation as for a correction class, everything is clear.” Nothing of the kind, it seems like such a simple school example, but there are so many VERY important conclusions! Here's another one:

You should strive to complete any task in the most rational way. If only because it saves time and nerves, and also reduces the likelihood of making a mistake.

If in a problem in higher mathematics you come across a system of two linear equations with two unknowns, then you can always use the substitution method (unless it is indicated that the system needs to be solved by another method). Not a single teacher will think that you are a sucker and will reduce your grade for using the “school method” "
Moreover, in some cases it is advisable to use the substitution method with a larger number of variables.

Example 2

Solve a system of linear equations with three unknowns

A similar system of equations often arises when using the so-called method of indefinite coefficients, when we find the integral of a fractional rational function. The system in question was taken from there by me.

When finding the integral, the goal is fast find the values ​​of the coefficients, rather than using Cramer’s formulas, the inverse matrix method, etc. Therefore, in this case, the substitution method is appropriate.

When any system of equations is given, first of all it is desirable to find out whether it is possible to somehow simplify it IMMEDIATELY? Analyzing the equations of the system, we notice that the second equation of the system can be divided by 2, which is what we do:

Reference: the mathematical sign means “from this follows that” and is often used in problem solving.

Now let's analyze the equations; we need to express some variable in terms of the others. Which equation should I choose? You probably already guessed that the easiest way for this purpose is to take the first equation of the system:

Here, no matter what variable to express, one could just as easily express or .

Next, we substitute the expression for into the second and third equations of the system:

We open the brackets and present similar terms:

Divide the third equation by 2:

From the second equation we express and substitute into the third equation:

Almost everything is ready, from the third equation we find:
From the second equation:
From the first equation:

Check: Substitute the found values ​​of the variables into the left side of each equation of the system:

1)
2)
3)

The corresponding right-hand sides of the equations are obtained, thus the solution is found correctly.

Example 3

Solve a system of linear equations with 4 unknowns

This is an example for you to solve on your own (answer at the end of the lesson).

Solving the system by term-by-term addition (subtraction) of the system equations

When solving systems of linear equations, you should try to use not the “school method”, but the method of term-by-term addition (subtraction) of the equations of the system. Why? This saves time and simplifies calculations, however, now everything will become clearer.

Example 4

Solve a system of linear equations:

I took the same system as in the first example.
Analyzing the system of equations, we notice that the coefficients of the variable are identical in magnitude and opposite in sign (–1 and 1). In such a situation, the equations can be added term by term:

Actions circled in red are performed MENTALLY.
As you can see, as a result of term-by-term addition, we lost the variable. This, in fact, is what the essence of the method is to get rid of one of the variables.

Using this mathematical program, you can solve a system of two linear equations with two variables using the substitution method and the addition method.

The program not only gives the answer to the problem, but also provides a detailed solution with explanations of the solution steps in two ways: the substitution method and the addition method.

This program can be useful for high school students in general education schools when preparing for tests and exams, when testing knowledge before the Unified State Exam, and for parents to control the solution of many problems in mathematics and algebra. Or maybe it’s too expensive for you to hire a tutor or buy new textbooks? Or do you just want to get your math or algebra homework done as quickly as possible? In this case, you can also use our programs with detailed solutions.

In this way, you can conduct your own training and/or training of your younger brothers or sisters, while the level of education in the field of solving problems increases.

Rules for entering equations

Any Latin letter can act as a variable.
For example: \(x, y, z, a, b, c, o, p, q\), etc.

When entering equations you can use parentheses. In this case, the equations are first simplified. The equations after simplifications must be linear, i.e. of the form ax+by+c=0 with the accuracy of the order of elements.
For example: 6x+1 = 5(x+y)+2

In equations, you can use not only whole numbers, but also fractions in the form of decimals and ordinary fractions.

Rules for entering decimal fractions.
The integer and fractional parts in decimal fractions can be separated by either a period or a comma.
For example: 2.1n + 3.5m = 55

Rules for entering ordinary fractions.
Only a whole number can act as the numerator, denominator and integer part of a fraction.
The denominator cannot be negative.
When entering a numerical fraction, the numerator is separated from the denominator by a division sign: /
The whole part is separated from the fraction by the ampersand sign: &

Examples.
-1&2/3y + 5/3x = 55
2.1p + 55 = -2/7(3.5p - 2&1/8q)

Solve system of equations

It was discovered that some scripts necessary to solve this problem were not loaded, and the program may not work.
You may have AdBlock enabled.
In this case, disable it and refresh the page.

JavaScript is disabled in your browser.
For the solution to appear, you need to enable JavaScript.
Here are instructions on how to enable JavaScript in your browser.

Because There are a lot of people willing to solve the problem, your request has been queued.
In a few seconds the solution will appear below.
Please wait sec...

If you noticed an error in the solution, then you can write about this in the Feedback Form.
Do not forget indicate which task you decide what enter in the fields.

Our games, puzzles, emulators:

A little theory.

Solving systems of linear equations. Substitution method

The sequence of actions when solving a system of linear equations using the substitution method:
1) express one variable from some equation of the system in terms of another;
2) substitute the resulting expression into another equation of the system instead of this variable;

$$ \left\( \begin(array)(l) 3x+y=7 \\ -5x+2y=3 \end(array) \right. $$

Let's express y in terms of x from the first equation: y = 7-3x. Substituting the expression 7-3x into the second equation instead of y, we obtain the system:
$$ \left\( \begin(array)(l) y = 7-3x \\ -5x+2(7-3x)=3 \end(array) \right. $$

It is easy to show that the first and second systems have the same solutions. In the second system, the second equation contains only one variable. Let's solve this equation:
$$ -5x+2(7-3x)=3 \Rightarrow -5x+14-6x=3 \Rightarrow -11x=-11 \Rightarrow x=1 $$

Substituting the number 1 instead of x into the equality y=7-3x, we find the corresponding value of y:
$$ y=7-3 \cdot 1 \Rightarrow y=4 $$

Pair (1;4) - solution of the system

Systems of equations in two variables that have the same solutions are called equivalent. Systems that do not have solutions are also considered equivalent.

Solving systems of linear equations by addition

Let's consider another way to solve systems of linear equations - the addition method. When solving systems in this way, as well as when solving by substitution, we move from this system to another, equivalent system, in which one of the equations contains only one variable.

The sequence of actions when solving a system of linear equations using the addition method:
1) multiply the equations of the system term by term, selecting factors so that the coefficients of one of the variables become opposite numbers;
2) add the left and right sides of the system equations term by term;
3) solve the resulting equation with one variable;
4) find the corresponding value of the second variable.

Example. Let's solve the system of equations:
$$ \left\( \begin(array)(l) 2x+3y=-5 \\ x-3y=38 \end(array) \right. $$

In the equations of this system, the coefficients of y are opposite numbers. By adding the left and right sides of the equations term by term, we obtain an equation with one variable 3x=33. Let's replace one of the equations of the system, for example the first one, with the equation 3x=33. Let's get the system
$$ \left\( \begin(array)(l) 3x=33 \\ x-3y=38 \end(array) \right. $$

From the equation 3x=33 we find that x=11. Substituting this x value into the equation \(x-3y=38\) we get an equation with the variable y: \(11-3y=38\). Let's solve this equation:
\(-3y=27 \Rightarrow y=-9 \)

Thus, we found the solution to the system of equations by addition: \(x=11; y=-9\) or \((11;-9)\)

Taking advantage of the fact that in the equations of the system the coefficients for y are opposite numbers, we reduced its solution to the solution of an equivalent system (by summing both sides of each of the equations of the original system), in which one of the equations contains only one variable.

Books (textbooks) Abstracts of the Unified State Examination and the Unified State Examination tests online Games, puzzles Plotting graphs of functions Spelling dictionary of the Russian language Dictionary of youth slang Catalog of Russian schools Catalog of secondary educational institutions of Russia Catalog of Russian universities List of tasks